CAT 2020 Set-2 | Question: 74

Question

From an interior point of an equilateral triangle, perpendiculars are drawn on all three sides. The sum of the lengths of the three perpendiculars is $s.$ Then the area of the triangle is 

• A. $\frac{\sqrt{3}s^{2}}{2}$
• B. $\frac{s^{2}}{\sqrt{3}}$
• C. $\frac{2s^{2}}{\sqrt{3}}$
• D. $\frac{s^{2}}{2 \sqrt{3}}$

Answer 1

Let the side of the equilateral triangle be $x.$

Let us consider an equilateral $\triangle \text{ABC, I}$ be interior point such that, $\text{ID, IE,}$ and $\text{IF}$ are perpendicular to sides $\text{BC,  AC,}$ and $\text{AB}$ respectively.



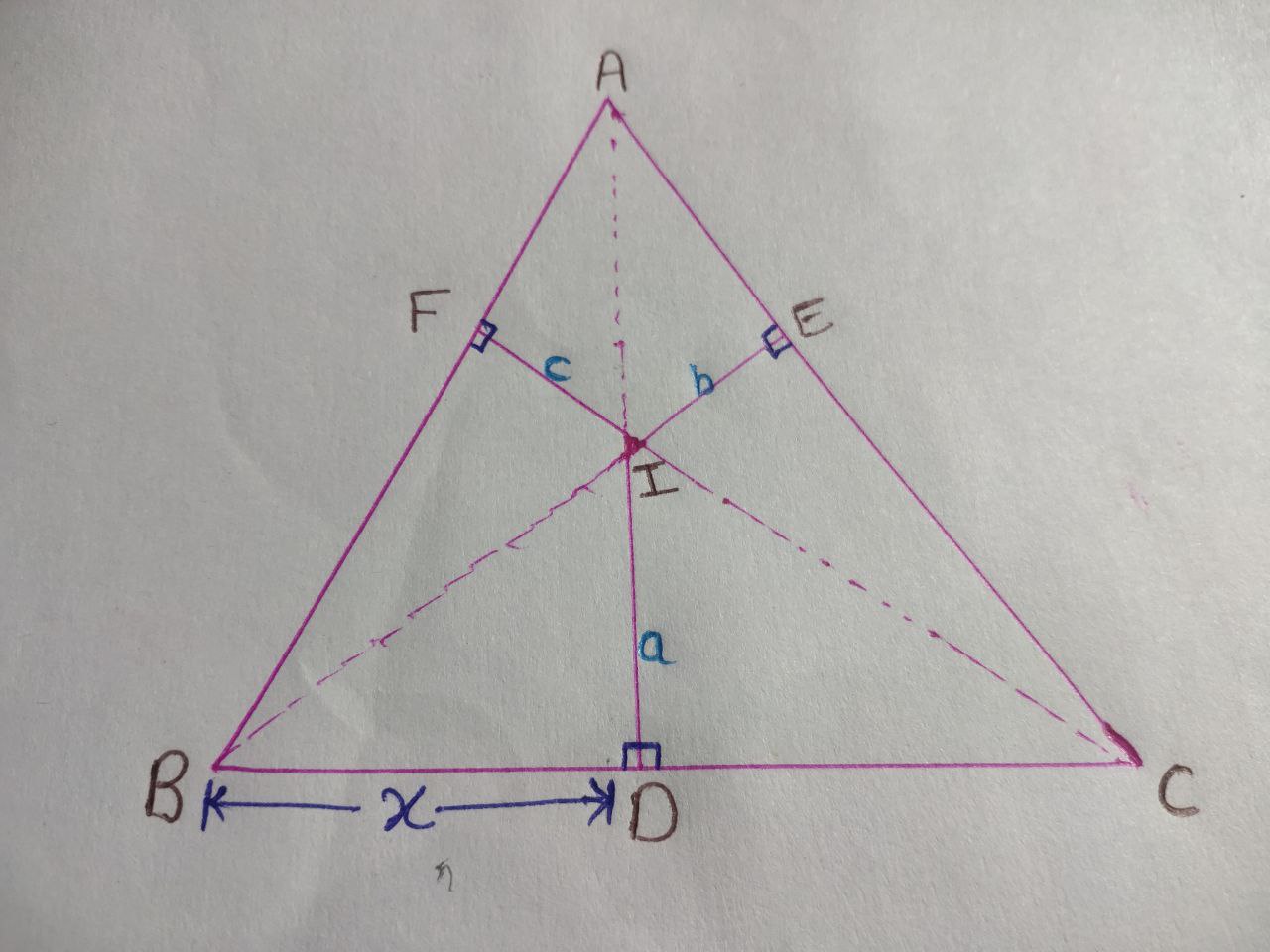
And, let $ \text{ID}= a, \text{IE}= b, \text{IF}= c.$

Also, $a+b+c = c$

Now, $ \text{Area of ABC} = \text{area of} \; \triangle \text{AIC} + \text{area of} \; \triangle \text{BIC} + \text{area of} \; \triangle \text{AIB}.$

$ \qquad \qquad = \frac{1}{2} \times x \times b + \frac{1}{2} \times x \times a + \frac{1}{2} \times x \times c $

$\qquad \qquad = \frac{1}{2} \times x (a+b+c) \; [\because a+b+c = s]$

$\qquad \qquad = \dfrac{xs}{2} \quad \longrightarrow (1)$

We know that, area of equilateral triangle $ = \dfrac{\sqrt{3}}{4} \times \text{(side)}^{2}$

Now, $ \dfrac{\sqrt{3}}{4} \times x^{2} = \dfrac{xs}{2} \quad [\because \text{From equation (1)}]$

$ \Rightarrow \boxed{ x = \frac{2s}{\sqrt{3}}}$

$\therefore$ The area of an equilateral triangle $ = \dfrac{\sqrt{3}}{4} \times x^{2}$

$\qquad \qquad = \dfrac{\sqrt{3}}{4} \times \left( \dfrac{2s}{\sqrt{3}} \right)^{2}$

$\qquad \qquad = \dfrac{\sqrt{3}}{4} \times \dfrac{2s}{\sqrt{3}} \times \dfrac{2s}{\sqrt{3}}$

$\qquad \qquad = \dfrac{s^{2}}{\sqrt{3}} \; \text{unit}^{2}.$

Correct Answer $: \text{B}$