The figure below shows two concentric circles with centre O. PQRS is a square inscribed in the outer circle. It also circumscribes the inner circle, touching it at points B, C, D and A. What is the ratio of the perimeter of the outer circle to that of polygon ABCD?
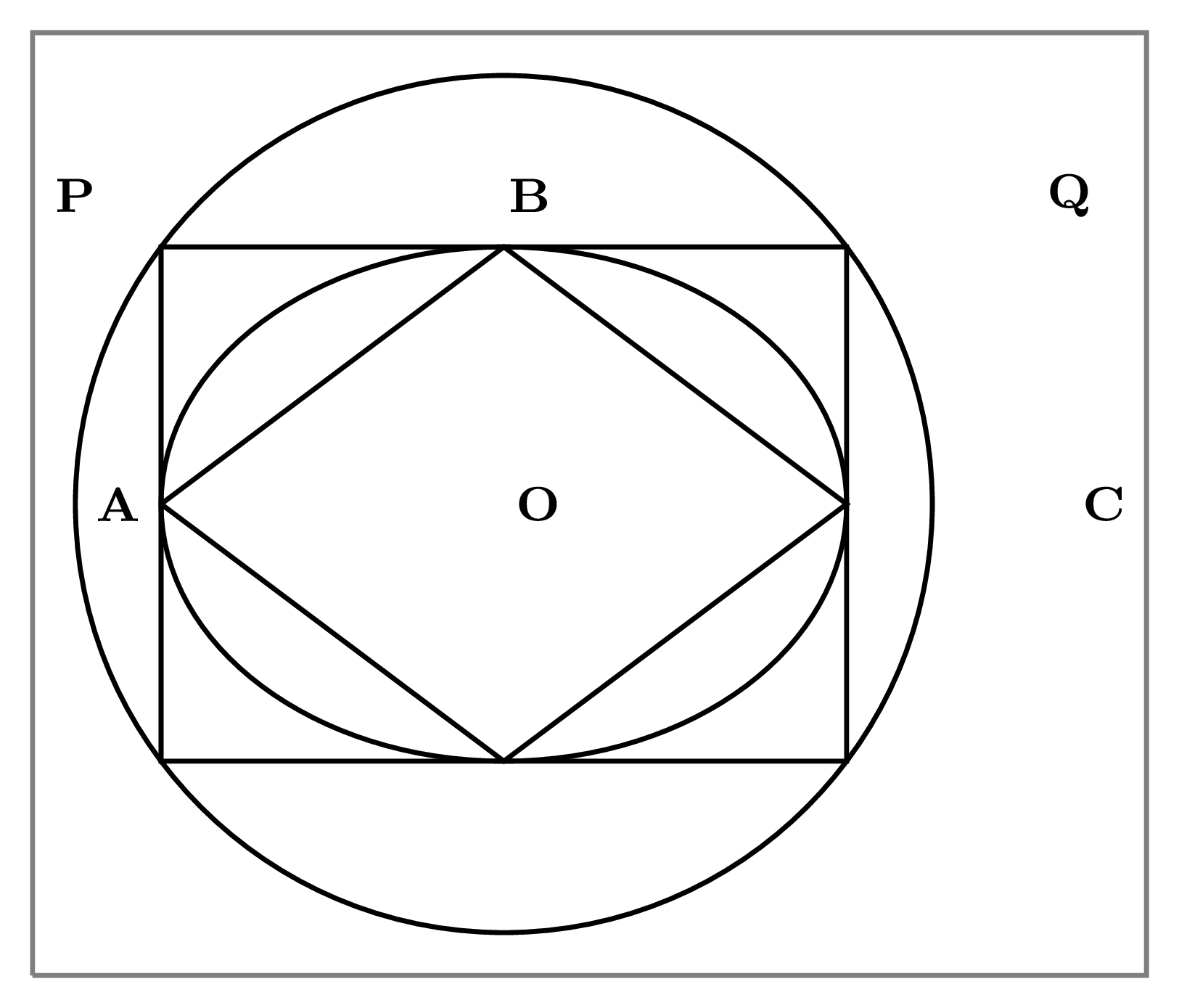
- $\frac{\pi}{4}$
- $\frac{3\pi}{2}$
- $\frac{\pi}{2}$
- ${\pi}$