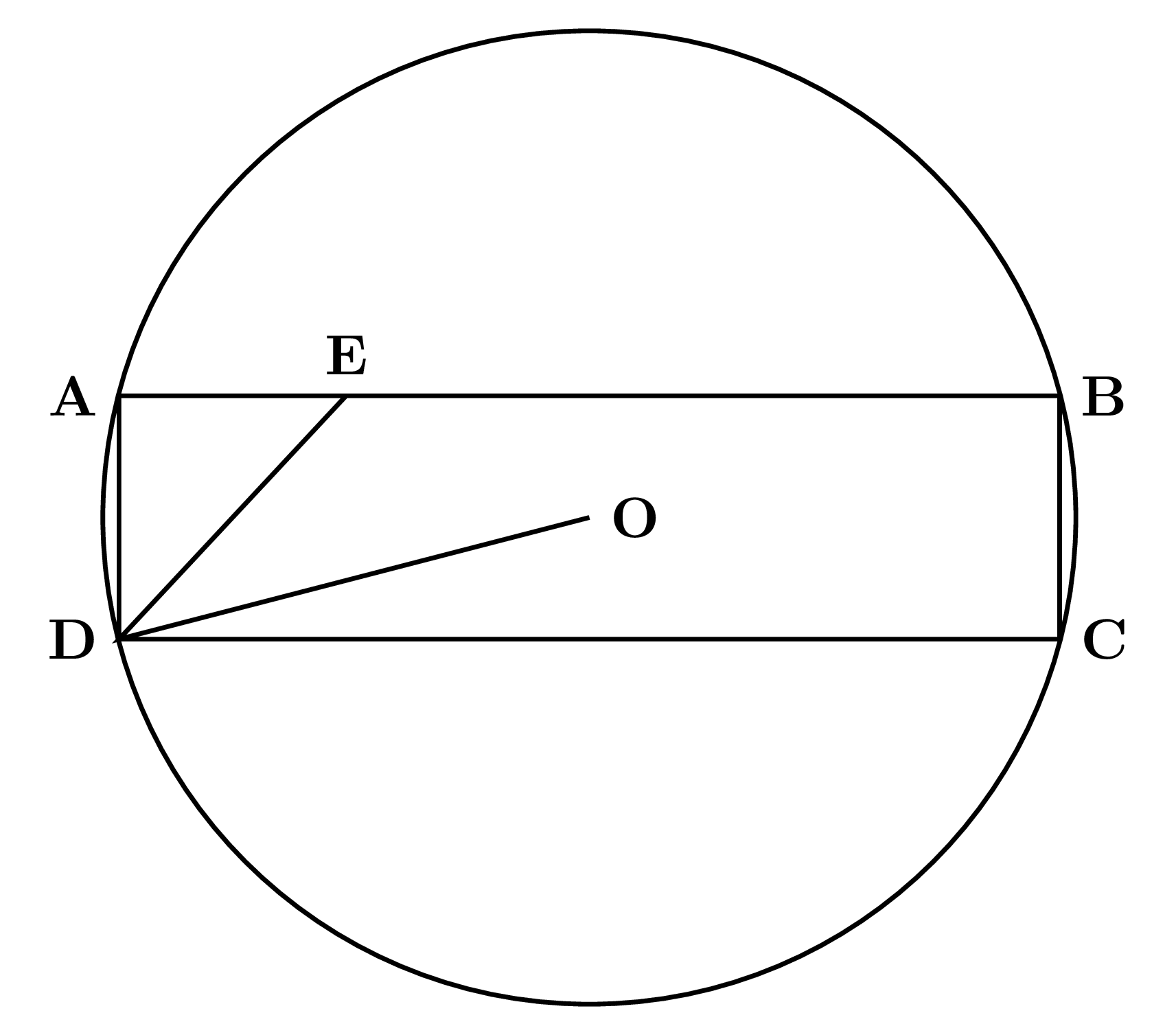
In the figure below (not drawn to scale), rectangle $\text{ABCD}$ is inscribed in the circle with centre at $\text{O}.$ The length of side $\text{AB}$ is greater than that of side $\text{BC}.$ The ratio of the area of the circle to the area of the rectangle $\text{ABCD}$ is $\pi: 3.$ The line segment $\text{DE}$ intersects $\text{AB}$ at $\text{E}$ such that $\measuredangle \text{ODC} = \measuredangle \text{ADE}.$ What is the ratio $\text{AE : AD}?$
- $1:\sqrt{3}$
- $1:\sqrt{2}$
- $1:2\sqrt{3}$
- $1:2$