Answer the question on the basis on the basis of the information given below.
Consider three circular parks of equal size with centres at $A_1,\:\: A_2,\:\:$ and $A_3$ respectively. The parks touch each other at the edge as shown in the figure (not drawn to scale). There are three paths formed by the triangles $A_1, \: A_2, \: A_3,\: B_1, \: B_2, \: B_3$, and $C_1, \: C_2, \: C_3,$ as shown. Three sprinters A, B, and C begin running from points $A_1,\: B_1$ and $C_1$ respectively. Each sprinter traverses her respective triangular path clockwise and returns to her starting point.
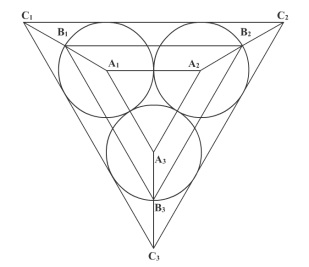
Sprinter A traverses distances $A_1 A_2, A_2 A_3,$ and $A_3 A_1$ at average speeds of 20, 30 and 15, respectively. B traverses her entire path at a uniform speed of $(10 \sqrt{3} + 20)$. C traverses distances $C_1 C_2, C_2 C_3$, and $C_3 C_1$ at average speeds of $\frac{40}{3} (\sqrt{3} +1)$, $\frac{40}{3} (\sqrt{3} +1)$, and 120 respectively. All speeds are in the same unit. Where would B and C be respectively when A finishes her sprint?
- $B_1, \: C_1$
- $B_3, \: C_3$
- $B_1, \: C_3$
- $B_1$, Somewhere between $C_3$ and $C_11$